CHE 423 Inorganic Chemistry Laboratory
Experiment #3: X-ray Diffraction
Introduction
Inorganic chemists, physicists, geologists, and materials scientists
use X-ray diffraction to determine molecular structures. Depending on the type of sample and the information
desired, a number of X-ray diffraction techniques are available: however, they
all depend on the same principles of diffraction. In this laboratory experience, you will learn how diffraction
works (Part A), explore the origin of the X-rays used in an instrument (Part
B), and determine the structure of a material from its powder diffraction
pattern (Part C). The lab report will
consist of a separate sheet of paper with your typed answers to the questions from
the various sections.
* Part A: Optical Diffraction Experiments
Purpose
To discover how a diffraction pattern is related to a repeating dot array; to use the diffraction pattern to measure the dimensions of the repeating dot array.
Introduction
Diffraction of a wave by a periodic array is due to phase differences that result in constructive and destructive interference (illustrated in Figure 1). Diffraction can occur when waves pass through a periodic array if the repeat distance of the array is similar to the wavelength of the waves. Observation of diffraction patterns when beams of electrons, neutrons, or X-rays pass through crystalline solids thus serves as evidence both for the wave nature of those beams and for the periodic nature of the crystalline solids. However, X-rays are hazardous and they require special detectors.
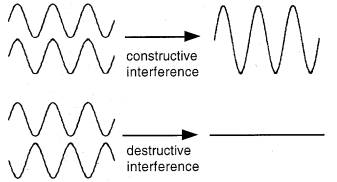
Figure 1. When waves line up (the oscillations are in phase), they add to give a bigger wave. When the peak of one wave is aligned with the trough of another, the waves annihilate each other.
Atoms, with spacings of about 10–10 m, require X-rays to create diffraction patterns. In this experiment, you make a change of scale. By using dots with spacings of about 10–4 m, visible light can be used instead of X-rays to create diffraction patterns. You will shine red laser light (633 nm wavelength) through a slide containing repeating arrays of dots, and observe Fraunhofer diffraction (see Figure 2).
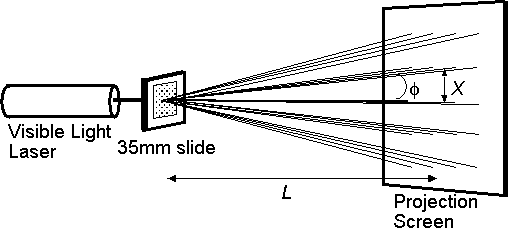
Figure 2. The Fraunhofer diffraction experiment.
Mathematically, the equations for Fraunhofer and Bragg diffraction (the basis of X-ray diffraction) are similar and embody the same functional dependence on the dot spacing (d), wavelength (l), and scattering angle (f or q), (see Figure 3).
In this experiment, you will first check how the size and orientation of the diffraction pattern is related to the periodic array that produced it, and then you will measure distances in diffraction pattern spacings in order to calculate the repeat distance for the array in the slide. By measuring the distances X and L, shown in Figure 2, and using the trigonometry definition that tan f = X/L, you can solve for f. Use of the Fraunhofer equation, d sin f = nl, then gives d when l is known.
Procedure
Obtain slides containing greatly reduced versions of arrays like those in Figure 4. Each photographic slide contains eight patches. Each patch has a different periodic array.
Look through a slide at a point source of white light. What do you see? Is the slide a diffraction grating? Why?
Shine a
He-Ne laser (633-nm wavelength) at a white piece of paper several meters
away. Fasten the laser in place. CAUTION:
The laser is potentially dangerous. Do
not look directly into a laser beam or shine a laser toward other people, as
damage to the eye can occur.
Look through a slide at the laser dot on the paper. What do you see? Why?
Put the slide in the laser beam and watch the paper. What happens? Light travels from the laser to the paper and then to your eye. Are the same results obtained if the beam goes through the slide before it hits the paper as after it hits the paper?
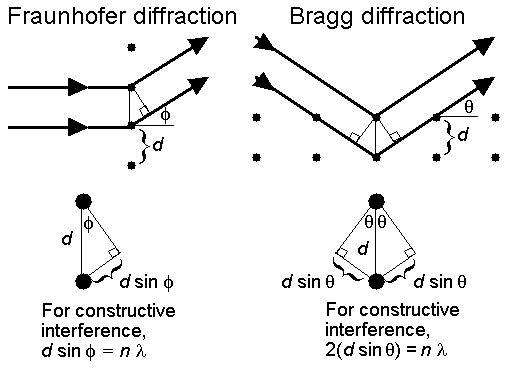
Figure 3. A comparison of Fraunhofer diffraction with Bragg diffraction. When waves are scattered by a periodic array, the path difference between any two waves must be a whole number of wavelengths, n, if the waves are to remain in phase and give constructive interference.
Pretend that you want to sketch the shape of the diffraction pattern from an array. What light source should you use (the white bulb or the laser)? Should you shine the beam through the slide or look at the spot through the slide? Pretend that you want to measure the spacing between the spots. What method is easiest?
Use a hand
lens to examine the arrays on the slide.
For ease of interpretation, you may prefer to keep a consistent
orientation for the slide.
Questions
Answer the following questions, devising and conducting experiments to obtain data where appropriate. “Data” for this experiment will sometimes consist of a sketch of an array and a sketch of the resulting diffraction pattern, and may include measurements of distances.
1. What is the diffraction pattern of a horizontal
array of lines? Of a vertical array of
lines?
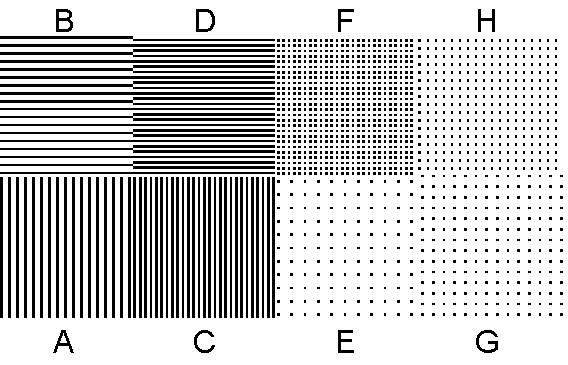
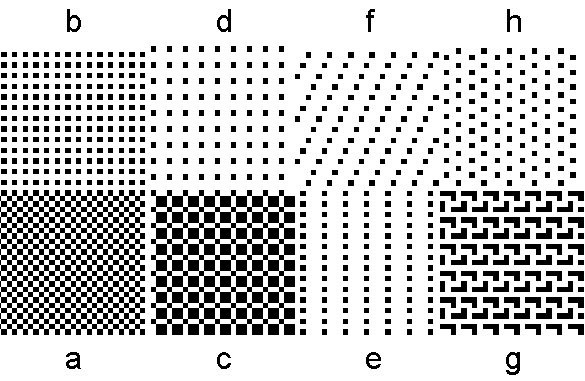
Figure 4. Arrays of dots that can be used to generate diffraction patterns with a laser. The actual patterns on the slide are much smaller.
2. What is the diffraction pattern of a square
array of dots? Of a rectangular array
of dots? Of a parallelogram array of
dots where the angle is not 90°? Of a
hexagonal array of dots? How do the
orientations of the diffraction patterns relate to the orientation of the array
of dots?
3. Find two similar arrays that differ only in
size. Does an array with a smaller
repeat distance give a diffraction pattern with a smaller repeat distance? Does an array with a larger repeat distance
give a diffraction pattern with a larger repeat distance?
4. Choose two arrays, carefully measure some
distances in the diffraction pattern, and calculate the size of the unit cell
for that array. What is the size in
millimeters of the spacing between dots in the array on the slides?
5. What happens if you put an additional dot in the array in the center of the unit cell? Does it matter if the dot placed in the center is the same size as those in the original array?
Part B: Making X-rays
Introduction
All X-ray instruments require a source of X-rays. One common way to generate X-rays is by sending an electron stream at a metal target. Using this technique, Mosely found in 1913 that each element emits X-rays of characteristic frequencies. His discovery contributed to our modern understanding of the electronic structure of the atom.
Procedure
View the demonstration of how X-rays are generated at the following Web site:
http://www.colorado.edu/physics/2000/xray/making_xrays.html
Questions
- Which type of X-rays—Bremsstrahlung or K-shell emission—is best for measuring X-ray diffraction and why?
- Describe the origin of the energy difference between Ka and Kb X-ray lines?
Part C: X-ray Powder Patterns
Introduction
Optical diffraction and X-ray diffraction are very similar. Using the understanding of reciprocal space that you learned in Part A will help you to understand the diffraction from a solid sample. The goal of this exercise is to be able to determine the structure of a cubic sample from the X-ray powder pattern.
Procedure
View the following pages from this Web site:
http://www.matter.org.uk/diffraction/x-ray/default.htm
- X-ray Diffraction
- X-ray Methods
- Bragg’s Law (pp 2-7)
- The Powder Method
- Indexing a Powder Pattern
- Indexing: Exercises
Questions
Answer the questions on each Web page. Include your answers in the report for this lab. For the final exercise, just index one sample.
Powder X-ray Diffraction Data Sheet
Sample No. _________
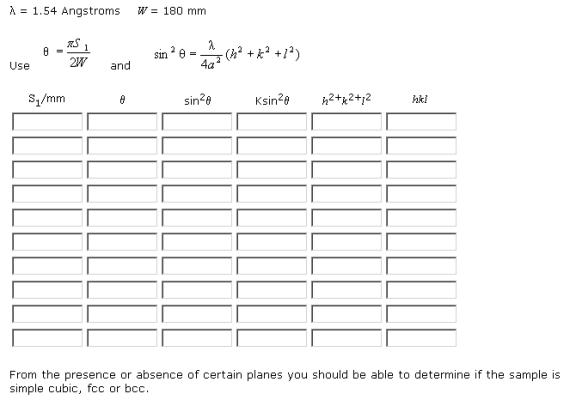
(from http://www.matter.org.uk/diffraction/x-ray/powder_analysis_data_sheet.htm)